Nonlinear Design. Bezier curves
| Bezier curves |
|
In the 20th century, however, this deficiency proved to be a real obstacle, especially in industrial design where complex curvilinear lines and surfaces must be defined precisely so as to be uniformly reproduced in metal or plastic. When you think of curvilinearity in technics, it's likely that one of the first things that comes to mind are the smooth contours of modern cars. Indeed it was the French Rénault car manufacturing corporation for which Pierre Bézier developed, in 1960s, a new design tool based on one very versatile variety of mathematical curves. These curves, named Bezier curves after their inventor, are now familiar to any user of a vector drawing program. The most commonly used Bezier curves of third order are fully defined by four points: two endpoints and two control points that do not lie on the curve itself but define its shape (Fig. 1). |
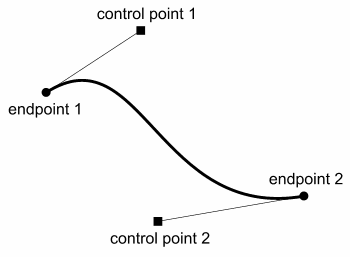
| Fig. 1: The anatomy of a Bezier curve |
| This simple construction is surprisingly versatile. A Bezier curve may be smooth and streamlined, but it may have very sharp turns and even fractures; it may have one or two bends, and even form loops (Fig. 2). It is also very capable at approximating other types of curves. For example, while you cannot draw an absolutely exact circle with Bezier curves, you can approximate a quarter of a circle (i.e. a 90o arc) by a Bezier with an error of less than 0.06%, which is certainly negligible for most practical cases. |
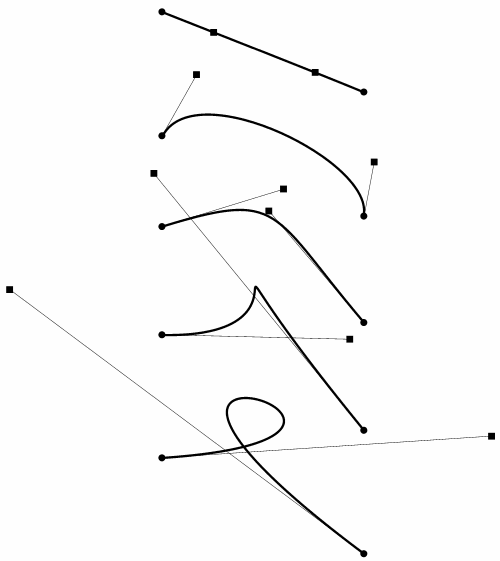
| Fig. 2: Exercising a Bezier |
| Now, Bezier curves are used almost exclusively for creating curvilinear shapes in all fields of design, from purely technical plans and blueprints to the most creative artistic genres. Their mathematical elegance is worth an article of its own; but for now, let's explore their creative capabilities from a visual, non-mathematical point of view. In the next sections, we'll try to discover the laws governing the world of Bezier curves, in the hope that these laws can, at least partially, explain their beauty and expression and provide directions for successfully using them in design. |
Revised: Feb. 12, 1999
URL: https://www.webreference.com/dlab/9902/bezier.html


 Find a programming school near you
Find a programming school near you