3D Animation Workshop: Lesson 53: Nendo - Curves Without Splines

Lesson 53 - Nendo--Curves Without Splines - Part 1

I just can't stop playing around with the new Nendo modeler. And the more I play with it, the more important it seems to me.

The intuitive feel and elegant design of this program are going to change the expectations of 3D artists. And the $100 price for something this powerful and professional is really going to change some thinking. But there are some special features that are so brilliant and innovative that they will make us reconsider some basic modeling practices. By the way, a free demo version of Nendo is available for download from Nichimen Graphics. This version does everything except export out to other programs, so it's a fantastic way of trying the program out. Frankly, I think this is required stuff for anyone serious about 3D. A warning, though. Although very inexpensive, Nendo's impressive interface requires good OpenGL graphics support.

Those fantastic heads modeled in Nendo on the Nichimen Graphics Web site really got me thinking. So much subtle curvature! How can you do this with straight polygonal modeling tools? Nendo has no splines at all, and really couldn't have them consistent with the closed-solid polygonal concept on which it's premised. This would seem to be a major limitation, because true spline modeling tools (or the quasi-spline MetaNURBS tools found in Lightwave) would seem necessary for creating smoothly curving surfaces. But Nendo has implemented some remarkable approaches for creating curvature without splines, and to test them I decided to model a wine glass.

Making a wine glass using true spline tools is a straightforward business. Here, using 3D Studio MAX, we draw a spline representing the profile of the object, including the inside surface.

This 2D object is then revolved 360 degrees to create the finished 3D model, a process called lathing in MAX and many other programs.

An angle view shows that the lathed object is composed of 16 polygonal segments around the circumference. This is probably the minimum number to simulate a circle for a low-poly version, though it would have to be much higher for high-resolution work.

Smoothly curving surfaces require a regular structure of rows and columns. The vertical columns of quadrangles around the circumference are equal and their number is determined directly by the user. But the horizontal rows of quads are unequal in height and their number is determined by the application so as to preserve the curvature of the underlying spline. Look at this wireframe view. Note the considerable density of rows at the bottom generated by the small curvature there.

Just because we are working in quads doesn't mean that we can ignore the final triangulated result. Unlike the head model in the previous lesson, in which contours were defined by edges between individual triangles, our regular pattern of quads is preserved by a regular pattern of diagonal edges. Don't be confused in this wireframe by the crossing of the diagonals from the inside and outside surfaces. Each quad is cut by only one diagonal.

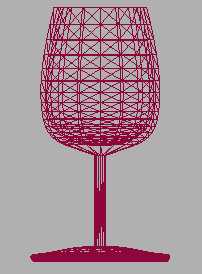
The total here is 1680 triangles, which is not all that bad for a smoothing curving object. So how would we do this is Nendo?

To Continue to Parts 2 and 3, Use Arrow Buttons

Created: Nov. 10, 1998
Revised: Nov. 10, 1998

URL: https://webreference.com/3d/lesson53/